"Exploring Rational Functions: Higher Order Polynomials, Asymptotes, and Holes"

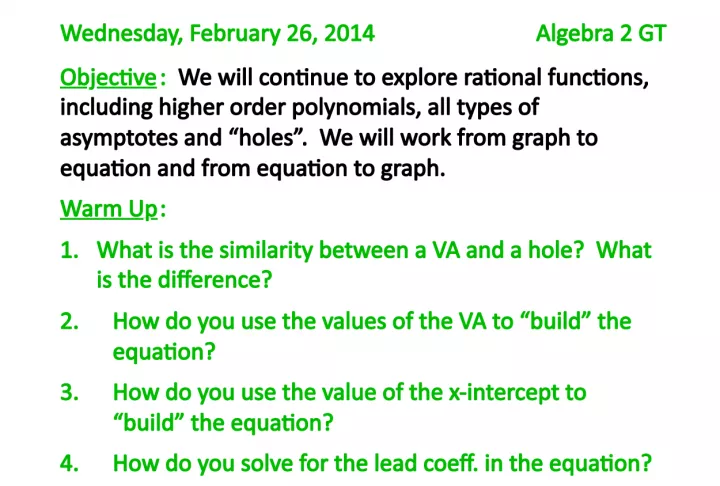
Slide1Wednesday, February 26, 2014                       Algebra 2 GT Objective :   We will continue to explore rational functions, including higher order polynomials, all types of asymptotes and “holes”.  We will work from graph to equation and from equation to graph. Warm Up : 1. What is the similarity between a VA and a hole?  What is the difference? 2. How do you use the values of the VA to “build” the equation? 3. How do you use the value of the x-intercept to “build” the equation? 4. How do you solve for the lead coeff. in the equation?

Slide2Weds. 2/26/14                       Algebra 2 GTComplete the CW worksheet and Study check out the “coolmath” website http://coolmath.com/algebra/23-graphing-rational- functions/index.html Quiz on 9.3 on Thursday 2/27

Slide3steps for finding key features of a rational function andusing that information to Create the Graph 1. FACTOR  both the numerator and denominator, and REDUCE  if possible. 2. If a factor remains in the  numerator  then its root is the x-intercept   [ point : (x, 0)] 3. If a factor(s) remains in the  denominator  then its root is (are) the  VA   [ equation : x = #] 4. If a factor was  canceled  then its root is the  hole   [ point : (x, y) ] 5. Determine HA  [ equation : y = #] 6. Find the  y-intercept  by letting  x = 0  in the reduced equation  [ point : (0, y)] 7. Graph all of this information and then use your understanding of the behavior of these graphs to sketch. If necessary, you can use some table values to help.

Slide4http://coolmath.com/algebra/23-graphing-rational- functions/index.html

Slide5Determining Horizontal AsymptotesThe value of the H.A. is determined by comparing the highest degree of  the numerator with that of the denominator. 1. If numerator > denominator ( top-heavy fraction ), then there is  NO H.A .  More on this next year. 2. If numerator < denominator ( bottom-heavy fraction ), then the H.A. is ALWAYS at  y  = 0 . 3. If numerator = denominator ( powers-equal fraction ), then the H.A. is ALWAYS at the line with equation  y = a/b , where a and be are the lead coefficients of the num. and denom.

Slide7(0, -1)

Slide9x = -3 y  = 5

Slide10We have a new parent function!  The RECIPROCAL FUNCTION:

Slide14Can you recreate this graph on yourgraphing calculator?

Slide15Variation Vocabulary …INVERSE Variation  – A relationship between variables characterized by the equation DIRECT Variation  – A relationship between variables characterized by the equation Constant of Variation  – the value of  k  (also, the slope of a line with  y -intercept = 0)

Slide16JOINT Variation – when one quantity varies directly with respect to two or more other quantities. COMBINED Variation  involves multiple variations. Some Translations: “ z  varies jointly with  x  and  y ” “ z  varies jointly with  x  and  y  and inversely with the square of  w ” “ z  varies directly with  x  and inversely with the product  wy ”

Slide17Common Sense understanding of Variation …Direct Variation – as one quantity increases, so does the other, by a constant amount. For example, as the amount of  time you drive increases , the  distance you drive also increases .  The constant of variation is the rate (speed) at which you are driving.

Slide18Common Sense understanding of Variation …Inverse Variation – as one quantity increases, the other decreases. For example, as the outside  temperature increases , the amount of  time  it takes an ice cube  to melt decreases .

Slide19Example:A quantity  c  varies jointly with  d  and the square of  g . Given  c = 30  when  d = 15  and  g = 2 , find  k , the constant of variation. Then, find  d  when  c = 6  and  g = 8 .