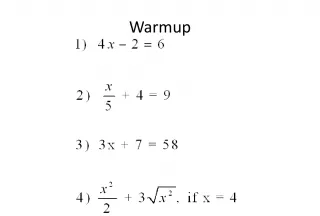Holt CA Course 1111: Solving Two Step Equations

Slide1Holt CA Course 111-1 Solving Two-Step Equations Warm  Up Warm  Up California  Standards California  Standards Lesson  Presentation Lesson  Presentation Preview Preview

Slide2Holt CA Course 111-1 Solving Two-Step Equations Warm Up Solve. 1.   n  + 9 = 17 2.  6 x  = 42 3.  71 –  z  = 55 4. n  = 8 x  = 7 z  = 16 = 9 y  = 72 y 8

Slide3Holt CA Course 111-1 Solving Two-Step Equations Preview of Grade 7          AF4.1   Solve two-step linear equations  and inequalities in one variable over the rational numbers , interpret the solution or solutions in the context from which they arose, and verify the reasonableness of the results.     California    Standards

Slide4Holt CA Course 111-1 Solving Two-Step Equations When you solve equations that have one operation, you use an inverse operation to isolate the variable. n  + 7  =  15 – 7        –7 n         =   8 2 x  + 3 = 23 – 3     – 3 2 x        = 20 Use the inverse of multiplying by 2 to isolate x.   x  = 10 You can also use inverse operations to solve equations that have more than one operation.

Slide5Holt CA Course 111-1 Solving Two-Step Equations Reverse the order of operations when solving equations that have more than one operation. Helpful Hint

Slide6Holt CA Course 111-1 Solving Two-Step Equations Solve. Check your answer. Additional Example 1A: Solving Two-Step Equations Using Division 9 c  + 3 = 39 9 c  + 3 = 39      – 3      –3 9 c        = 36 c  = 4 Subtract 3 from both sides. Divide both sides by 9. 9 c 9 = 36     9

Slide7Holt CA Course 111-1 Solving Two-Step Equations Check. Additional Example 1A Continued 9 c  + 3 = 39 9 (4)  + 3 39 ? = ? = ? = 36 + 3    39 39    39 Substitute 4 for c. 4 is a solution.

Slide8Holt CA Course 111-1 Solving Two-Step Equations Solve. Additional Example 2: Solving Two-Step Equations Using Multiplication A. 6 + y 5 = 21 y 5 =  21 6 + – 6                –6 y 5 = 15 y 5 =  (5) 15 (5) y  = 75 Subtract 6 from both sides. Multiply both sides by 5.

Slide9Holt CA Course 111-1 Solving Two-Step Equations Jamie rented a canoe while she was on vacation. She paid a flat rental fee of $85.00, plus $7.50 each day. Her total cost was $130.00. For how many days did she rent the canoe? Additional Example 3:  Consumer Math Application Let  d  represent the number of days she rented the canoe. 7.5 d  + 85 = 130         – 85      –85 7.5 d        =   45 7.5 d  =   45 7.5 7.5 d   = 6 Jamie rented the canoe for 6 days. Subtract 85 from both sides. Divide both sides by 7.5.

Slide10Holt CA Course 111-1 Solving Two-Step Equations –6 m  – 8 = –50 –6 m  – 8 = –50        + 8       +8 –6 m        = –42 Add 8 to both sides. Solve. Check the answer. m  = 7 Divide both sides by –6. –6 m   –6   = –42       –6 Check It Out!  Example 1B

Slide11Holt CA Course 111-1 Solving Two-Step Equations Check It Out!  Example 2 Solve. A. 8 + y 2 = 48 y 2 = 48 8 + – 8               –8 y 2 = 40 y 2 =  (2) 40 (2) y  = 80 Subtract 8 from both sides. Multiply both sides by 2.

Slide12Holt CA Course 111-1 Solving Two-Step Equations Check It Out!  Example 3 Jack’s father rented a car while they were on vacation. He paid a rental fee of $20.00 per day, plus 20¢ a mile. He paid $25.00 for mileage and his total bill for renting the car was $165.00. For how many days did he rent the car? Let  d  represent the number of days he rented the car. 20 d  + 25 = 165       – 25      – 25 20 d         =  140 20 d  =   140 20   20 d   = 7 Jack’s father rented the car for 7 days. Subtract 25 from both sides. Divide both sides by 20.

Slide13Holt CA Course 111-1 Solving Two-Step Equations Lesson Quiz Solve. Check your answers. 1.  6 x  + 8 = 44 2.  14 y  – 14 = 28 3. 4.   y  = 3 x  = 6 63   =  m v  = –112 m 7 12 =     + 3 – 6 = 8 5.  Last Sunday, the Humane Society had a 3-hour     adoption clinic. During the week the clinic is open     for 2 hours on days when volunteers are available.     If the Humane Society was open for a total of 9     hours last week, how many weekdays was the     clinic open? 3 days v –8